1.
The amount at a later point in time is known as a __________
future value.
2.
__________
Compound (or Compounded) interest refers to earning interest on previously earned interest.
3.
The amounts contained in a future value of 1 table for various combinations of n and i are referred to as future value __________
factors.
4.
In the future value of 1 table, n refers to the number of __________
periods of time, such as months, quarters, years.
5.
If you know the future value of a single deposit, the interest rate, and the number of periods that the interest is compounded, you will be able to calculate the __________
present value by using a future value of 1 table.
6.
Assume that you are calculating the future value of a single deposit by using a future value of 1 table. The deposit will be invested in an account earning 12% per year for four years. If the interest will be compounded quarterly, the number of periods (n) will be __________
16 (4 years X 4 quarters per year) and i will be 3%.
7.
An unrestricted deposit of $1,000 grows to a future value of $5,000 through the compounding of interest. Under the accrual basis of accounting, the $4,000 of growth should be reported as __________
interest revenue (or interest income).
8.
Under the accrual basis of accounting, the interest earned over a three-year period on a single deposit should be reported on a company's income statement __________.
9.
Which compounding of an 8% annual interest rate will result in a larger future value?
10.
If you know the present value of a single amount, the future value of that amount, and the number of periods that the interest will be compounded, you can calculate the __________
interest rate.
11.
When using the future value of 1 table to calculate the future value of a deposit earning 10% per year compounded semiannually, you would use a factor from the column headed __________
5% (10% per year divided by 2 six-month periods per year).
12.
An account or deposit will earn 12% interest per year for two years. Assuming that you are using the future value of 1 table and the interest is compounded monthly, you will find the factor in the row where n is __________
24 (2 years X 12 months per year) periods.
13.
An account or deposit will earn 12% interest per year for two years. Assuming that you are using the future value of 1 table and that the interest is compounded monthly, you will find the factor in the column where i is __________
1% (12% divided by 12 months per year).
Use the following future value of 1 factors for solving the remaining questions:
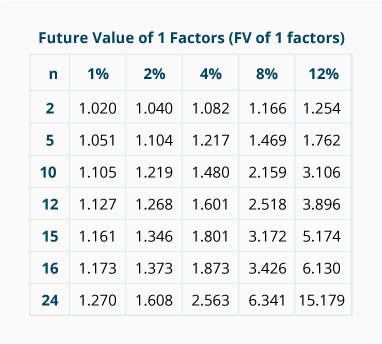
14.
A deposit of $1,000 on January 1, 2025 will have a future value of $__________
$1,469.
FV of 1 = PV x FV of 1 factor for n = 5 years, i = 8% compounded annually
FV of 1 = $1,000 x 1.469
FV of 1 = $1, 469 on December 31, 2029 (or January 1, 2030) if it is invested at 8% per year and the interest is compounded annually.
15.
A deposit of $10,000 on January 1, 2025 will have a future value of $__________
$14,800.
FV of 1 = PV x FV of 1 factor for n = 10 semiannual periods, i = 4% per semiannual period
FV of 1 = $10,000 x 1.480
FV of 1 = $14,800 on December 31, 2029 (or January 1, 2030) if it is invested at 8% per year and the interest is compounded semiannually.
16.
A deposit of $2,000 on January 1, 2025 will have a future value of $__________
$2,540.
FV of 1 = PV x FV of 1 factor for n = 24 months, i = 1% per month
FV of 1 = $2,000 x 1.270
FV of 1 = $2,540 on December 31, 2026 (or January 1, 2027) if it is invested at 12% per year and the interest is compounded monthly.
17.
A deposit of $1,000 on January 1, 2025 will have a future value of $__________
$1,373.
FV of 1 = PV x FV of 1 factor for n = 16 quarters, i = 2% per quarter
FV of 1 = $1,000 x 1.373
FV of 1 = $1,373 on December 31, 2028 (or January 1, 2029) if it is invested at 8% per year and the interest is compounded quarterly.
18.
The interest rate that is necessary for a deposit of $1,000 to grow to $5,474 after 15 years of interest compounded annually is __________
12%.
FV of 1 = PV x FV of 1 factor for n = 15 years, i = ??% compounded yearly;
$5,474 = $1,000 x FV of 1 factor for n = 15 yrs, i = ??% compounded yearly;
5.474 = FV of 1 factor for n = 15 yrs, i = ??% compounded yearly;
Look only in the row, n = 15, and locate the factor 5.474. It is in the column where i = 12%.% per year.
19.
The annual interest rate that is necessary for a deposit of $1,000 to grow to $1,373 after four years of interest compounded quarterly is __________
8%.
FV of 1 = PV x FV of 1 factor for n = 16 quarters, i = ??% per quarter;
$1,373 = $1,000 x FV of 1 factor for n = 16 qtrs, i = ??% per quarter;
1.373 = FV of 1 factor for n = 16 qtrs, i = ??% per quarter;
Look only in the row, n = 16, and locate the factor 1.373. It is in the column where i = 2%. This means that the rate per quarter is 2%. Since the question is asking for the annual rate, multiply the rate per quarter times 4 to arrive at 8% per year.% per year.
20.
The number of years required for an investment of $1,000 to grow to $2,563 when invested at an annual rate of 8% compounded semiannually is __________
12 years.
FV of 1 = PV x FV of 1 factor for n = ?? six-month periods, i = 4% semiannually;
$2,563 = $1,000 x FV of 1 factor for n = ?? six-month periods, i = 4% semiannually;
2.563 = FV of 1 factor for n = ?? six-month periods, i = 4% semiannually;
Look only in the column, i = 4%, and locate the factor 2.563. It is in the row where n = 24. This means that the number of six-month periods is 24, and that equals 12 years. years.
